Back to
Tohsuke Urabe Laboratory
|
Back to Tohsuke Urabe Laboratory |
Consider a plane. Move it and put it onto exactly the same plane. (We admit turning it over, but don't admit folding it.) We call such movement a transformation and the set of all such transformations of the plane is called a Euclidean group, which is denoted by E.
Note that NOT moving the plane is a kind of movement and is a special
transformation. We therefore give it its own name. We call it an identity
transformation.
Theorem
Any element of the Euclidean group E is one of the following:
Consider a line on the plane and fix it as the axis. The transformation such that turning over the plane by rotating it through 180 degrees about the axis is called a reflection on this line. We regard the line as a mirror, and it associates any point on the plane with the mirror image of that point. Intuitively, a reflection "flips" the plane across the axis.
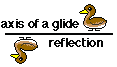
The transformation equivalent to performing a reflection on a line and
a translation parallel to the same line after the reflection is called a
glide reflection on this line.
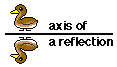
Now, assume that we have a repeating wallpaper pattern drawn on a plane. Consider the set of all elements in the Euclidean group, E, such that the pattern on the result of the transformation is exactly the same pattern as the original. We denote this subset of E by G. We call G the wallpaper group of this pattern.
(In Japanese this is called "monyo gun." The word "gun" means "group" in English, while "monyo" means "patterns on traditional clothes." In Japan, we have a rich collection of traditional patterns that are used to decorate our clothes, i.e. "ukata patterns." One hundred or more years ago, the Japanese were dressed in much more elaborately designed, patterned clothes. However, we have very few kinds of patterned wallpapers.)
Consider the case where G satisfies the following two conditions:
Condition (1) means that the pattern is repeated in at least two directions.
For the simplest stripes, G has a translation with an arbitrarily short
moving distance in the direction of the stripes. However, most repeating
patterns satisfy both (1) and (2). From a mathematical point of view, cases
satisfying both (1) and (2) are the most interesting. Cases where either
(1) or (2) are not satisfied are considered separately, therefore, we assume
conditions (1) and (2) are both satisfied below.
Under this assumption, group G coincides with one of seventeen kinds seen on the previous page.
Let us explain how to distinguish the seventeen kinds:
The above table may be difficult to understand, but by using it we can
decide the unique type of seventeen symmetry types for any given pattern
satisfying the conditions (1) and (2). Please check that the example
on the previous page satisfies conditions in the above table.
Another guide might help to decide the type of symmetry. It's helpful to think in terms of a lattice. First, pick an origin point, O, on the plane. (You can pick any point.) The subset of all points on the plane obtained by moving O with translations belonging to G is called the lattice of G. (Note that we are considering only translations in G.) Actual lattices such as window bars or jail bars have many horizontal or vertical bars equidistant from each other. These intersecting points are similar to the mathematical concept called a lattice. Likewise, if we make a pattern by tiling with one kind of tile with all of them oriented in the same direction and putting a mark on every tile at the same point, then the set of all the marks on the completed pattern is the lattice. Picking a characteristic point in the pattern as the origin O, we can easily obtain the lattice.
A general lattice is all intersecting points in the checkered pattern of parallelograms. In some cases it has a characteristic shape. We refer to them by the names below.
| general lattice |  |
rectangular lattice | 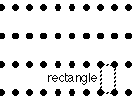 |
| square lattice | 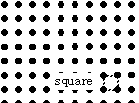 |
hexagonal lattice | 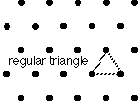 |
| centered lattice |  |
For a centered lattice, as in the figure above, we always choose an axis
of reflection and consider it the standard direction together with the lattice.
We agree that a square is a kind of a rectangle, and a rectangle is a kind
of a parallelogram. We have the following theorem:
Theorem
By using the concept of lattices and by the above theorem you can easily choose several candidates of seventeen symmetry types. Thereafter, you can decide the unique type by close consideration.
We have explained you the essentials of the mathematical theory of wallpaper patterns. You can improve your understanding further by checking the theory for many concrete examples aroud you. We have prepared an examination for you here.
|
|
|
|
Back to Tohsuke Urabe Laboratory |
|